Author:
(1) K. Razi Naqvi, Department of Physics, Norwegian University of Science and Technology (NTNU), 7094 Trondheim, Norway
Table of Links
3 Comments elicited by the solution to Milne’s problem for B-particles
6 Concluding remarks and References
4 Flux to a spherical trap
For absorption of R-particles by a spherical trap of radius 𝑅, Ziff found the “Milne extrapolation length” to be ≈ 0.29795219𝑙 for 0 < 𝑙 ≤ 2𝑅. Since this result contradicts (at least at first sight) a great deal of work on closely related problems, including some carried out by the present author, and no resolution was offered in Ziff’s paper [20], or in two of its sparkling sequels [21, 22], a probe into the discrepancy is warranted.


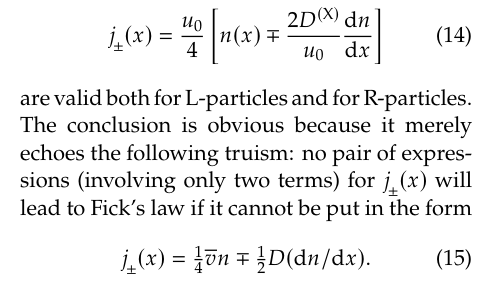
We are thus led to the seemingly insipid but sublimely conciliatory conclusion that the expressions
4.1 The boundary condition at the wall
 4.2 The boundary condition for a spherical sink
4.2 The boundary condition for a spherical sink
Citing four articles by Collins and co-workers, Ziff makes two remarks, stating, first, that the “constant 𝛾 [the linear extrapolation length] must be determined by empirical arguments”, and next, that “to lowest order they [meaning various prescriptions by Collins et al] generally give the same result 𝛾 = (𝑙/3)[1 + 𝒪(𝜀)], . . . ”, where 𝜀 ≡ 𝑙/𝑅. I am unable to see an unequivocal statment to this effect in any of the four papers cited by Ziff (his refs. 15–18). In their Eqs. (3) and (4), reproduced here in my notation, Frisch and Collins [23] provide the clearest statement of their BC:
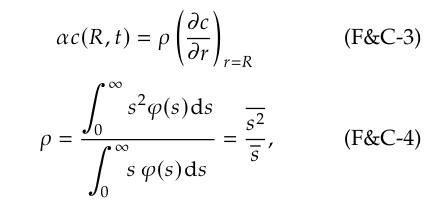
Since the 𝛼-dependence is wrong (see below) and we are interested in a black sphere, we will set 𝛼 = 1 in Eq. (F&C-3). By examining ref. 15 of Ziff, a 1949 article [24], one can convince oneself that Eq. (F&C-4) should be changed to

but a general statement about 𝜌 (which is to be identified with the linear extrapolation length for a black sphere) is still beyond reach because the “jumps” above discussed can be taken essentially as the path of the molecule between successive collisions. The persistence of velocity upon collision, however, causes the jump density function 𝜑(𝑠) to be no longer spherically uniform but to depend upon the direction of the jump immediately preceding the jump under consideration in the manner of a Markov process. However, . . . this effect can be accounted for by multiplying ⟨𝑠 2 ⟩ by a correction factor slightly greater than unity. For convenience in this discussion, it will be assumed that this correction factor has been already incorporated in ⟨𝑠 2 ⟩ and in the other moments of 𝜑(𝑠).
For the two cases of interest to us, namely L-particles and R-particles, we get 𝜌 = 2ℓ/3 and 𝜌 = 𝑙/3, in agreement with the calculations based on the plane symmetric system.
It is worth adding here that the jump model presented in the above cited 1949 article [24] can be shown to imply the following boundary condition for a grey sphere:
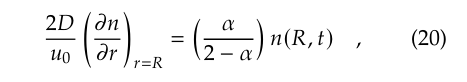
but the inference did not appear in print until 1982 [7].
4.3 The case of a small sphere: the Achilles heel of the “Pearson-Rayleigh random walker”
So far, we have not come across any conclusion (emerging from Ziff’s model) that cannot be reconciled with its counterparts from inverse and regular Brownian motion. However, Ziff’s calculations revealed the extrapolation length 𝛾 to be independent of 𝑙/𝑅 for 0 < 𝑙/𝑅 ≤ 2, in sharp contrast to the results found in neutron transport studies, which have been summarized by Sahni [25] andWilliams [26], both of whom have presented their own calculations as well. When the Brownian analog of this problem was investigated, the coagulation rate constant showed an umistakable dependence on the value of Λ/𝑅 [27–29]; an important conclusion that emerged from these investigations is worth stressing yet again: when moment methods are used for solving the KKE, attempts to obtain better results by increasing the number of moments will prove to be futile, because, beyond a certain order, convergence is lost.
This paper is available on arxiv under CC BY 4.0 DEED license.

